TIP4P/2005 model of water

The TIP4P/2005 model is a re-parameterisation of the original TIP4P potential for simulations of water. TIP4P/2005 is a rigid planar model, having a similar geometry to the Bernal and Fowler model.

Parameters

$r_{\mathrm {OH} }$ (Å)	$\angle$HOH , deg	$\sigma$ (Å)	$\epsilon /k$ (K)	q(O) (e)	q(H) (e)	q(M) (e)	$r_{\mathrm {OM} }$ (Å)
0.9572	104.52	3.1589	93.2	0	0.5564	-2q(H)	0.1546

• DL_POLY FIELD file for the TIP4P/2005 model
• GROMACS files for the TIP4P/2005 model

Phase diagram

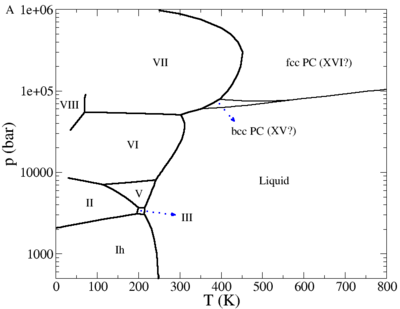
The phase diagram of the TIP4P/2005 model is given in a publication by Abascal, Sanz and Vega and for negative pressures in the publication

Plastic crystal phases

Recent simulations have suggested the possibility of a plastic crystal phase or phases for water

Surface tension

The surface tension has been studied for the TIP4P/2005 model

Self-diffusion coefficient

The TIP4P/2005 potential has a self-diffusion coefficient, in bulk water at 298 K, of 0.21 Ų ps⁻¹ in a classical simulation of 216 water molecules (experimental value: 0.23 Ų ps⁻¹) .

Shear viscosity

The shear viscosity for the TIP4P/2005 model is 0.855 mPa.s at 298 K and 1 bar (experimental value 0.896 mPa.s ).

References

Related reading

• Helena L. Pi, Juan L. Aragones, Carlos Vega, Eva G. Noya, Jose L. F. Abascal, Miguel A. Gonzalez and Carl McBride "Anomalies in water as obtained from computer simulations of the TIP4P/2005 model: density maxima, and density, isothermal compressibility and heat capacity minima", Molecular Physics 107 pp. 365-374 (2009)

External links and resources

• Presentation: Comparing the performance of TIP4P/2005 with other water models