Hard spherocylinders: Difference between revisions
mNo edit summary |
Carl McBride (talk | contribs) m (Added mention of a plastic crystal phase.) |
||
| Line 2: | Line 2: | ||
The '''hard spherocylinder''' model consists of an impenetrable cylinder, capped at both ends | The '''hard spherocylinder''' model consists of an impenetrable cylinder, capped at both ends | ||
by hemispheres whose diameters are the same as the diameter of the cylinder. The hard spherocylinder model | by hemispheres whose diameters are the same as the diameter of the cylinder. The hard spherocylinder model | ||
has been studied extensively because of its propensity to form both [[Nematic phase | nematic]] and [[Smectic phases | smectic]] [[Liquid crystals | liquid crystalline]] phases <ref>[http://dx.doi.org/10.1038/332822a0 D. Frenkel, H. N. W. Lekkerkerker and A. Stroobants "Thermodynamic stability of a smectic phase in a system of hard rods", Nature '''332''' p. 822 (1988)]</ref>. One of the first studies of hard spherocylinders was undertaken by Cotter and Martire <ref>[http://dx.doi.org/10.1063/1.1673232 Martha A. Cotter and Daniel E. Martire "Statistical Mechanics of Rodlike Particles. II. A Scaled Particle Investigation of the Aligned to Isotropic Transition in a Fluid of Rigid Spherocylinders", Journal of Chemical Physics '''52''' pp. 1909-1919 (1970)]</ref> using [[scaled-particle theory]], and one of the first simulations was in the classic work of Jacques Vieillard-Baron <ref>[http://dx.doi.org/10.1080/00268977400102161 Jacques Vieillard-Baron "The equation of state of a system of hard spherocylinders", Molecular Physics '''28''' pp. 809-818 (1974)]</ref>. In the limit of zero diameter the hard spherocylinder becomes a line segment, often known as the [[3-dimensional hard rods |hard rod model]]. | has been studied extensively because of its propensity to form both [[Nematic phase | nematic]] and [[Smectic phases | smectic]] [[Liquid crystals | liquid crystalline]] phases <ref>[http://dx.doi.org/10.1038/332822a0 D. Frenkel, H. N. W. Lekkerkerker and A. Stroobants "Thermodynamic stability of a smectic phase in a system of hard rods", Nature '''332''' p. 822 (1988)]</ref> as well as forming a [[Plastic crystals | plastic crystal]] phase for short <math>L</math> <ref>[http://dx.doi.org/10.1063/1.474626 C. Vega and P. A. Monson "Plastic crystal phases of hard dumbbells and hard spherocylinders", Journal of Chemical Physics '''107''' pp. 2696-2697 (1997)]</ref> . One of the first studies of hard spherocylinders was undertaken by Cotter and Martire <ref>[http://dx.doi.org/10.1063/1.1673232 Martha A. Cotter and Daniel E. Martire "Statistical Mechanics of Rodlike Particles. II. A Scaled Particle Investigation of the Aligned to Isotropic Transition in a Fluid of Rigid Spherocylinders", Journal of Chemical Physics '''52''' pp. 1909-1919 (1970)]</ref> using [[scaled-particle theory]], and one of the first simulations was in the classic work of Jacques Vieillard-Baron <ref>[http://dx.doi.org/10.1080/00268977400102161 Jacques Vieillard-Baron "The equation of state of a system of hard spherocylinders", Molecular Physics '''28''' pp. 809-818 (1974)]</ref>. In the limit of zero diameter the hard spherocylinder becomes a line segment, often known as the [[3-dimensional hard rods |hard rod model]], and in the limit <math>L=0</math> one has the [[hard sphere model]]. | ||
==Volume== | ==Volume== | ||
The molecular volume of the spherocylinder is given by | The molecular volume of the spherocylinder is given by | ||
Revision as of 18:02, 30 November 2010
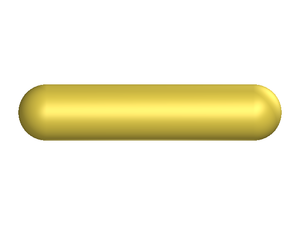
The hard spherocylinder model consists of an impenetrable cylinder, capped at both ends by hemispheres whose diameters are the same as the diameter of the cylinder. The hard spherocylinder model has been studied extensively because of its propensity to form both nematic and smectic liquid crystalline phases [1] as well as forming a plastic crystal phase for short [2] . One of the first studies of hard spherocylinders was undertaken by Cotter and Martire [3] using scaled-particle theory, and one of the first simulations was in the classic work of Jacques Vieillard-Baron [4]. In the limit of zero diameter the hard spherocylinder becomes a line segment, often known as the hard rod model, and in the limit one has the hard sphere model.
Volume
The molecular volume of the spherocylinder is given by
where is the length of the cylindrical part of the spherocylinder and is the diameter.
Minimum distance
The minimum distance between two spherocylinders can be calculated using an algorithm published by Vega and Lago [5]. The source code can be found here. Such an algorithm is essential in, for example, a Monte Carlo simulation, in order to check for overlaps between two sites.
Virial coefficients
- Main article: Hard spherocylinders: virial coefficients
Phase diagram
- Main aritcle: Phase diagram of the hard spherocylinder model
See also
References
- ↑ D. Frenkel, H. N. W. Lekkerkerker and A. Stroobants "Thermodynamic stability of a smectic phase in a system of hard rods", Nature 332 p. 822 (1988)
- ↑ C. Vega and P. A. Monson "Plastic crystal phases of hard dumbbells and hard spherocylinders", Journal of Chemical Physics 107 pp. 2696-2697 (1997)
- ↑ Martha A. Cotter and Daniel E. Martire "Statistical Mechanics of Rodlike Particles. II. A Scaled Particle Investigation of the Aligned to Isotropic Transition in a Fluid of Rigid Spherocylinders", Journal of Chemical Physics 52 pp. 1909-1919 (1970)
- ↑ Jacques Vieillard-Baron "The equation of state of a system of hard spherocylinders", Molecular Physics 28 pp. 809-818 (1974)
- ↑ Carlos Vega and Santiago Lago "A fast algorithm to evaluate the shortest distance between rods", Computers & Chemistry 18 pp. 55-59 (1994)
Related reading



