Radial distribution function: Difference between revisions
Carl McBride (talk | contribs) |
Carl McBride (talk | contribs) No edit summary |
||
| Line 1: | Line 1: | ||
The '''radial distribution function''' is a special case of the [[pair distribution function]] for an isotropic system. | The '''radial distribution function''' is a special case of the [[pair distribution function]] for an isotropic system. | ||
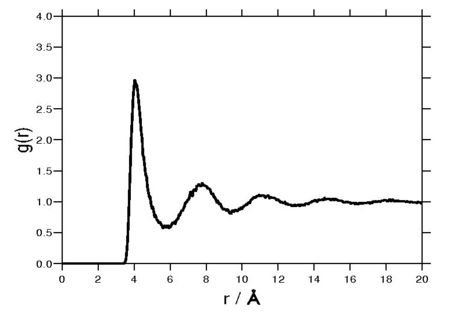
A [[Fourier analysis | Fourier transform]] of the radial distribution function results in the [[structure factor]], which is experimentally measurable. | A [[Fourier analysis | Fourier transform]] of the radial distribution function results in the [[structure factor]], which is experimentally measurable. The following plot is of a typical radial distribution function for the monatomic Lennard-Jones liquid. | ||
[[Image:LJ_rdf.png|center|450px|Typical radial distribution function for the monatomic Lennard-Jones liquid.]] | |||
==Density Expansion of the radial distribution function== | ==Density Expansion of the radial distribution function== | ||
The '''radial distribution function''' of a compressed gas may be expanded in powers of the density (Ref. 2) | The '''radial distribution function''' of a compressed gas may be expanded in powers of the density (Ref. 2) | ||
Revision as of 15:04, 3 October 2007
The radial distribution function is a special case of the pair distribution function for an isotropic system. A Fourier transform of the radial distribution function results in the structure factor, which is experimentally measurable. The following plot is of a typical radial distribution function for the monatomic Lennard-Jones liquid.
Density Expansion of the radial distribution function
The radial distribution function of a compressed gas may be expanded in powers of the density (Ref. 2)
where is the number of molecules per unit volume and is the intermolecular pair potential. The function is normalized to the value 1 for large distances. As is known, , , ... can be expressed by cluster integrals in which the position of of two particles is kept fixed. In classical mechanics, and on the assumption of additivity of intermolecular forces, one has
where is the distance , where is the Mayer f-function
and
References
- John G. Kirkwood and Elizabeth Monroe Boggs "The Radial Distribution Function in Liquids", Journal of Chemical Physics 10 pp. 394-402 (1942)
- B. R. A. Nijboer and L. Van Hove "Radial Distribution Function of a Gas of Hard Spheres and the Superposition Approximation", Physical Review 85 pp. 777 - 783 (1952)
- J. L. Lebowitz and J. K. Percus "Asymptotic Behavior of the Radial Distribution Function", Journal of Mathematical Physics 4 pp. 248-254 (1963)
- B. Widom "On the Radial Distribution Function in Fluids", Journal of Chemical Physics 41 pp. 74-77 (1964)














