Hard superball model: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
No edit summary |
||
| Line 2: | Line 2: | ||
A superball is defined by the inequality | A superball is defined by the inequality | ||
:<math>\frac{x | |||
:<math>\left|\frac{x}{a}\right|^{2q} + \left|\frac{y}{a}\right|^{2q} +\left|\frac{z}{a}\right|^{2q} \le 1</math> | |||
where ''x'', ''y'' and ''z'' are scaled Cartesian coordinates with ''q'' the deformation parameter, and we use radius a of the particle as our unit of length. The shape of the superball interpolates smoothly between two Platonic solids, namely the octahedron (''q'' = 0.5) and the cube (''q'' = ∞) via the sphere (''q'' = 1) as shown in the left figure. | where ''x'', ''y'' and ''z'' are scaled Cartesian coordinates with ''q'' the deformation parameter, and we use radius a of the particle as our unit of length. The shape of the superball interpolates smoothly between two Platonic solids, namely the octahedron (''q'' = 0.5) and the cube (''q'' = ∞) via the sphere (''q'' = 1) as shown in the left figure. | ||
Revision as of 20:58, 16 September 2012
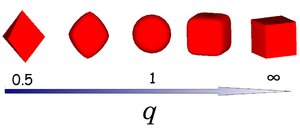
A superball is defined by the inequality
where x, y and z are scaled Cartesian coordinates with q the deformation parameter, and we use radius a of the particle as our unit of length. The shape of the superball interpolates smoothly between two Platonic solids, namely the octahedron (q = 0.5) and the cube (q = ∞) via the sphere (q = 1) as shown in the left figure.
